Globoidal indexing cams, globoidal indexers

Globoidal indexing cams are a special application of globoidals cams and a special type of indexing mechanism.

Globoidal indexers are very compact and stiff, and the stepping motion of the follower (the wheel that holds the rollers) is freely configurable with harmonic, jerk-free motions within wide limits. By adjusting the center distance, the gear backlash can be optimized.

Globoidal indexing cam gears are very powerful and achieve high performance.

That is why they are very popular in practice.

Just by changing the motion design, the same type of gear can also be used for other applications, eg for tool changer systems, as a pre-gearbox for achieving synchronized operation or for energy optimization.

Our software OPTIMUS MOTUS contains powerful and field-proven components for designing, calculating and simulating globoidal cam gearboxes and generating NC and manufacturing data.

Make use of our experience with globoidal indexing cams!

Skip to ...

• Variants
• Design
• Spreading
• Freecutting
• Roundings
• Chamfers
• Track concatenation
• NC Optimization
• Calculation
• Software

To the homepage of  Nolte NC-Kurventechnik GmbH

Variants

Globoidal indexers come in different versions:

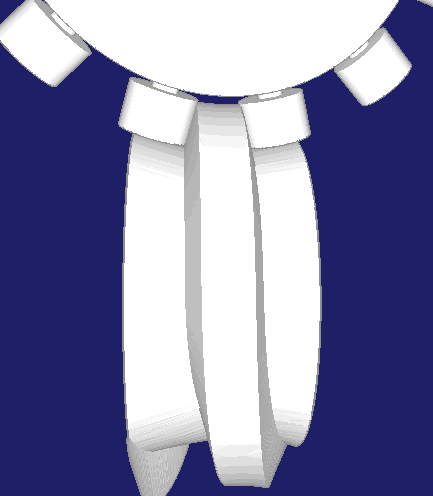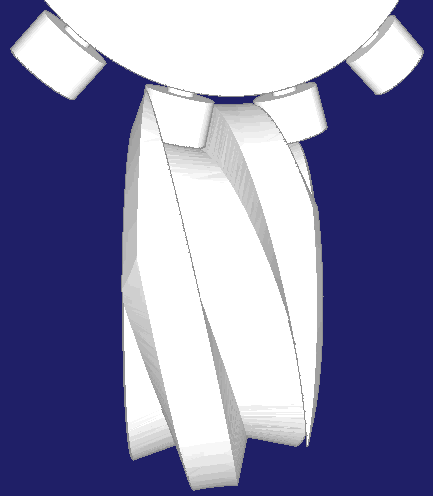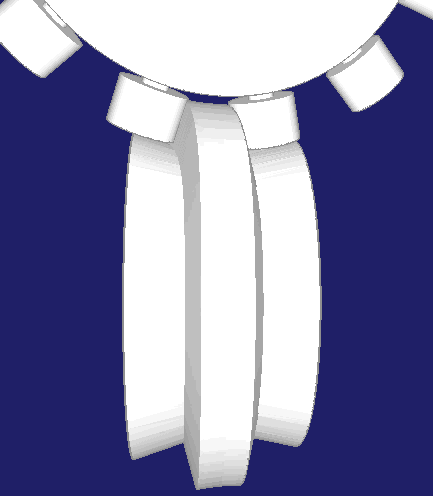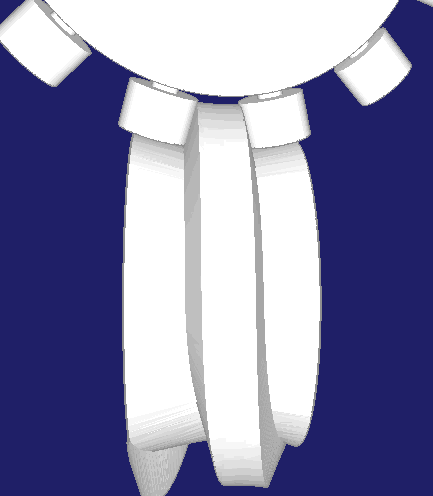
Groove / ridge in the middle

A standard indexing cam gear contains dwells in the movement sequence. The follower does one step and then remains for a while in standstill. A distinguishing feature of globoidal indexers is where the rollers are in the dwell phase. Theoretically, the roller wheel (follower) can assume its dwell position in any angular position relative to the cam. The rule, however, is that the rollers are then arranged symmetrically.

One possibility for this is to put the dwell area of the cam groove in the middle, as in this animation:

Much more common but is the arrangement in which the dwell bulge between two rollers is centered, as here:

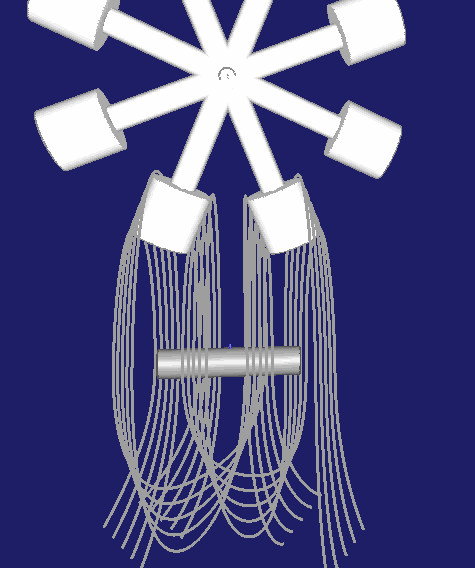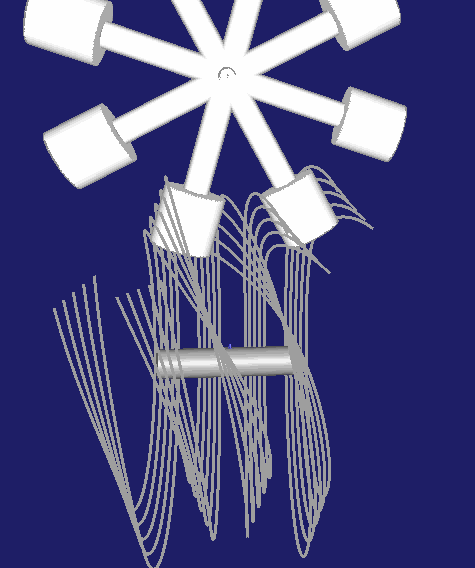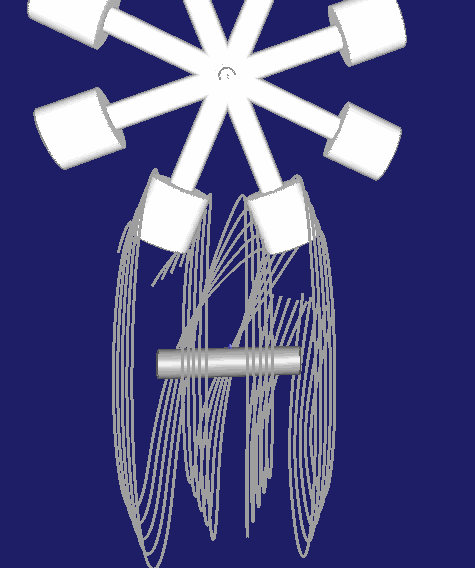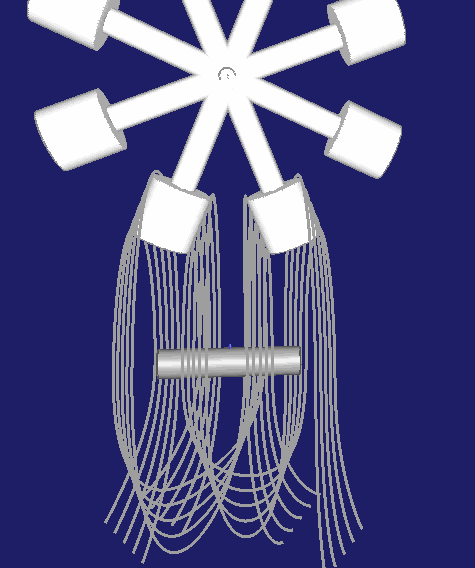
The advantage of this second arrangement is that the cam gear backlash can be adjusted via the center distance between camshaft and follower rotation axis.

An essential task of the indexer is to securely fix the follower with the turntable in the dwell, so that the products can be processed reproducibly on the turntable. During the motion phase, great slack would lead to shocks and thus to wear. It is often even optimal to slightly tension the rollers with the cam over the center distance.

This clearance setting via the axial distance is made possible by the fact that the roller axis and thus the contact line between roller and cam is not perpendicular to the cam axis of rotation. If you change the distance between the cam rotation axis and the follower pivot point, the rollers also move away from or towards the cam flank.

Track count

Another distinguishing feature of the standard designs is the number of different tracks, needed. The track count corresponds to the number of indexes covered by one cam rotation.

The previous two animations show step gears (indexers) with one track each.

Here is an illustration for a two-track globoidal indexer:

Finally, a representation for a three-track globoid indexing cam:

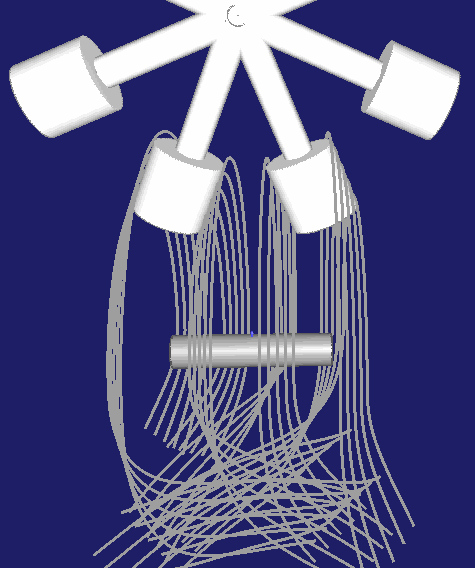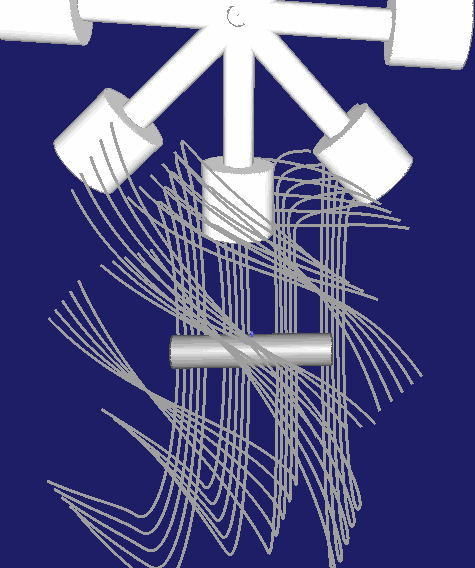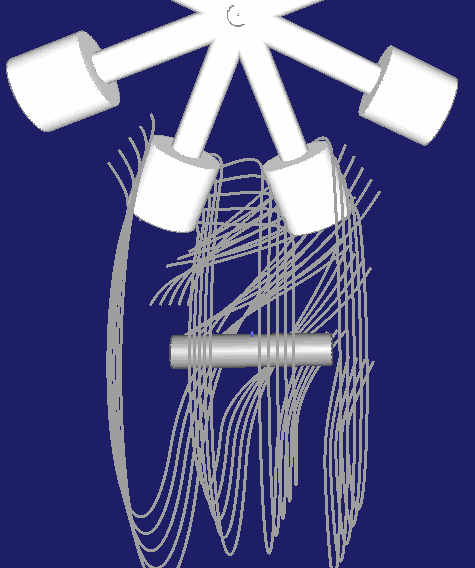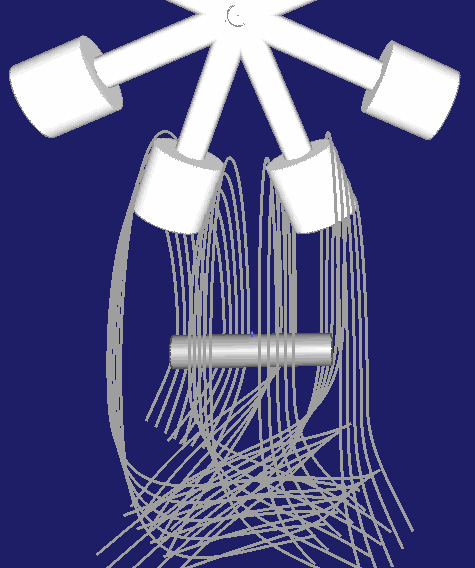
Step / Pilgrim / Continuous motion

The standard globoidal indexer transforms a continuous drive rotation into a stepwise output rotation, ie sequences of indexing and dwell. A particular advantage of globoidal indexing cams, for example, compared to the parallel-cam indexers is that the output rotation may also run backwards to the same extent.

Thus, in a globoidal cam gearbox, also an oscillating output motion is possible, even with a large output rotation angle as in the following animation:

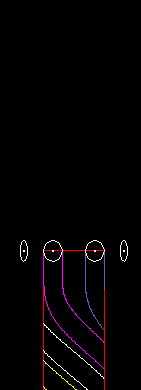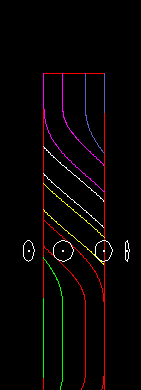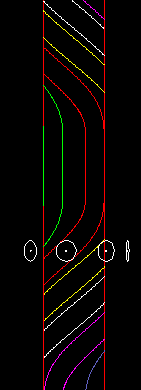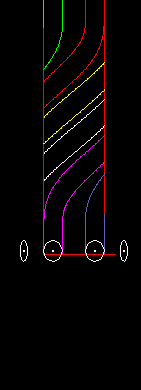
Also conceivable are pilgrim step movements, that is to say continuous rotations, but with backward steps. Cams of this type are used for tool change facilities:

Furthermore, globoidal cam gears with a constant ratio between input and output rotation are common. You can have quite large gear ratios in one stage with that.

The kinematic concept of the globoidal cam gear leaves a lot of freedom in the design of the output movements. Forward and backward very large output movements are possible.

In addition to the step movements, the oscillating movements, the constant gear ratios and the pilgrim movements, also very general output movements without standstill are possible. For example, the driving motion of a linkage could be designed to achieve a specific law of motion or constant speed on the output of the linkage. Then the globoidal cam modifies the transmission of the linkage in an appropriate way to generate the right overall transmission function.

Our OPTIMUS MOTUS software supports all of these design approaches!

Special designs

We can modify and calculate globoid indexers in some more ways with our software, eg with

• Camber / inclination of the rollers on the follower
• Non-uniform roller division in multi-track cams
• Inserting the grooves on the inside of a cam ring instead of the outer case
• Tilting of the follower plane with respect to the cam axis of rotation
• Use conical or extremely spherical rollers

Back to top

Design

In the sales and project planning of indexing drives, a quick statement is needed as to whether an envisaged globoidal indexing drive will last for a given load and at the given speed, and what life span is expected.

It is equally interesting which gear size is required for the demanded durability for a given load and given speed, or whether an indexing gear for the design parameters specified by the customer is even available.

The user or customer simply wants to know whether he can get a suitable indexer for his transport application, and how expensive it will be.

For these questions, our software OPTIMUS MOTUS has a special design module for indexing cams.

We also use this software for service calculations.

In a dialog box you enter the basic data for the cam calculation, eg center distance, cam width, roller pitch radius, number of rollers, roller diameter, roller crowning radius, dynamic load rating of the roller, step angle, indexing angle, flank height, working height, turntable mass moment of inertia, cam speed.

OPTIMUS MOTUS calculates the gearbox and evaluates kinematically and dynamically whether the gearbox is executable and which nominal service life the rollers and the cam can achieve. It also takes into account that possibly multiple rollers in contact share the load!

In particular, the following values are determined:

Coverage (Overlap distance)

Minimum run length over which several cam flanks are engaged. The overlap distance should be greater than 0, otherwise the cam can not completely control the follower. The turntable then may have great slack.

Ridge (bulge) thickness

The smallest width of the bulges between the cam grooves on the cam case, in the flank center and in the groove bottom. If the ridges are too thin, they can not carry the load.

Transmission angle

The minimum transmission angle is a measure of the slope of the camd path. Theoretically, 90 degrees is the optimal value, but achievable only at 0 degree step angle, not as a design goal. Following the general recommendations, 45 degrees is a good, 60 degrees a very good value for the minimum transmission angle. Ultimately, the size of the gearbox depends on which minimum transmission angle you want to allow.

Profile radius of curvature

The minimum profile radius of curvature for the convex cam profile regions is determined at the outer edge of the cam flank, in the middle and in the groove base. Theoretical values below 0 mm indicate an undercut. The theoretical cam flank undercuts itself there. If a small positive profile radius of curvature is achieved on the contact level between roller and cam, the cam design is generally acceptable. The smallest radius of curvature is influenced by a number of design parameters.

The OPTIMUS MOTUS software automatically replaces undercut regions in the exported 3D data with roundings to give you a chance to extrude the roller track volume (the space of a cam groove) in CAD.

Maximum occurring roller force

The largest contact force between roller and cam.

Maximum drive power

The maximum value of the required drive power in kW.

Roller lifetime

Particularly interesting for the design is the nominal roller life in hours. This is the life span statistically achieved by 90% of all bearings at the assumed loads and cycle times, not regarding operating factors. However, if many rollers are seated on the follower, the entire follower already fails with the first damaged roller, and it is far more likely that any of N rollers will fail than a specific, previously selected roller. Therefore, a nominal roller life is calculated for the entire roller wheel.

Cam durability

Hardness grades of 60 HRC or 62 HRC are typical for hardened cam flanks. Using the maximum Hertzian stress calculated for the specified crowning radius, our software estimates the achievable cam flank life in terms of pitting.

Maximum output speed

The maximum angular speed at the follower during the indexing motion.

Maximum output acceleration

The maximum angular acceleration at the follower during the indexing motion.

In addition, the program estimates, with which maximum static load, with which maximum moment of inertia and at what speed the gearbox can be operated, how wide the cam must be at least and which straight line component is required in the law of motion.

All results are summarized in a compact and clear way.

Here is an example of such an evaluation:

Back to top

Spreading

Slack (backlash) is bad for gears of all kinds, especially at high speeds:

• With slack, the position of the output member is not defined. The gearbox does not fulfill its positioning task precisely.
• Slack leads to shocks between rollers and cam flanks or more generally between drive and follower. Impacts mean wear and noise. Bumpy loads are more destructive than controlled, harmonic loads.

For conjugate cams (also called double cams, complementary cams, main and counter cams, working and return cam), the residual backlash is often "pushed" out of the cam gear in practice, for example by eccentric bolts or other adjustments, to achieve the best possible running characteristics.

In globoidal indexing cams, however, eccentric bolts do not work. The slack can be adjusted with globoidal indexers by changing the center distance (see above). On the other hand, the center distance is the most important kinematic dimension of the gear box, and you do not really want to adjust it.

To take the backlash out of the indexer, the cam flanks can be provided in a controlled manner with allowance. The cam flanks are "spread". Thus, the rollers on the follower are pressed apart by a defined amount and in a controlled way.

Globoid indexing cams are produced in the form of one or more grooves, each with two cam flanks. If one of the flanks is offset to the outside, the counter flank must be taken back accordingly so that the roller will not be pinched. You can choose which flank should be retracted (the other one will be shifted outside then), so that the rollers can be pressed inwards as well as outwards.

By spreading, the rollers are braced against each other, and there will be additional static forces between cam and rollers. The larger the oversize and the stiffer the bearing, the greater the additional forces. Therefore, the allowance should be assumed as small as possible, just to compensate the maximum expected backlash from manufacturing and assembly.

The following animation illustrates the principle of spreading.

Shown is the developed view of a globoidal indexing cam for a constant gearing ratio.

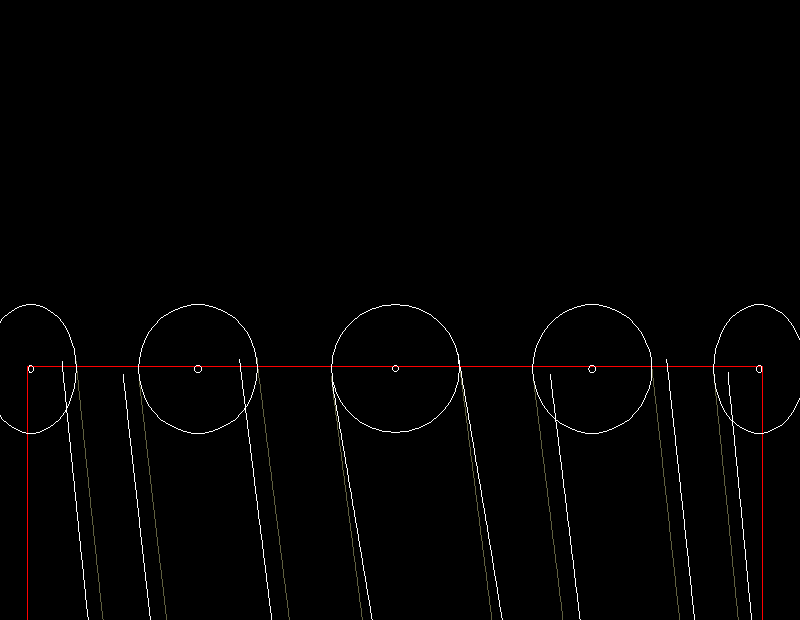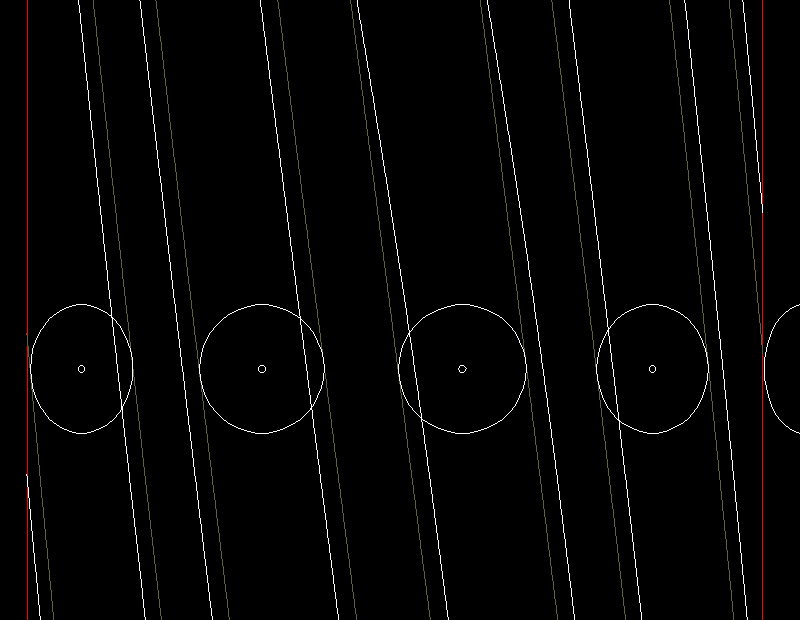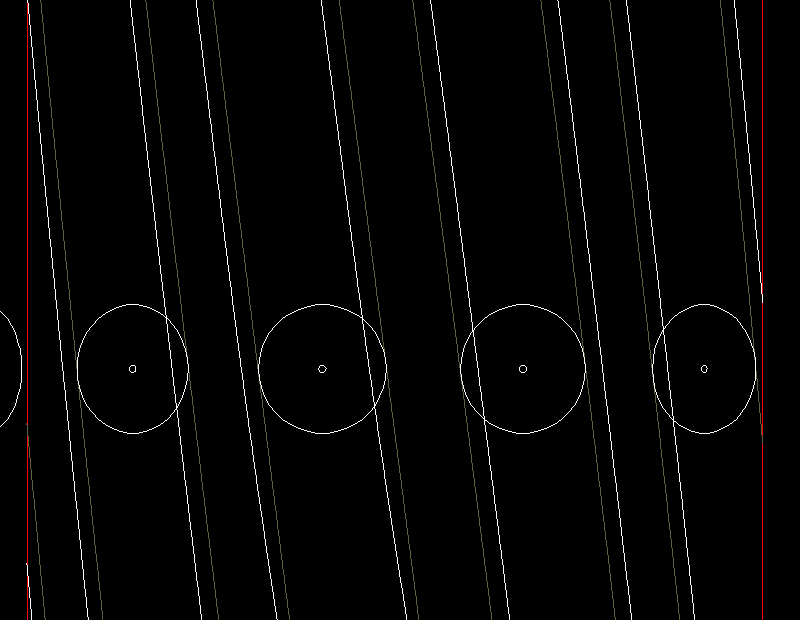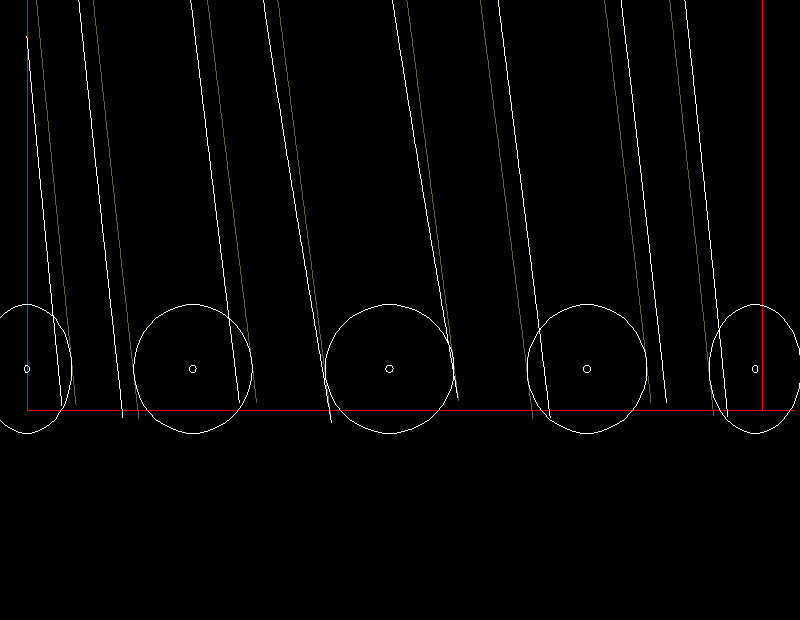
In gray, you see the theoretical cam flanks, and in white the actually calculated cam flanks, how they are to be produced. You can see that the cam rollers lie straight against the theoretical cam flanks but penetrate into the actually produced flanks. In the real gearbox, the cam flanks will then push the rollers outwards and clamp them against each other.

Small note: With the allowance, we have exaggerated here very much so that you can see the effect in the animation. In practice, you have very small offsets only.

For cylindrical indexing cam gears, the spreading function is equally applicable.  Here you will find a 3D animation to show spreading.

Back to top

Freecutting

When manufacturing and assembling cam gearboxes there will always be some small residual errors, for example, as regards the position of the rollers on the follower, the roller diameter, the angularity of the roller axes, the center distance, etc.

These deviations together with the offset from the spreading lead to the rollers not entering the cam body perfectly tangential to the camd path, but with a slight offset.

Assuming you cut the theoretically calculated grooves including spreading offsets, but without further adaptation, the rollers would hit the edges when entering the cam body. In the developed view, these are the acute-angled corners between left and right front side and the camd paths. These shocks cause noise, wear and overall reduce roller durability.

If you know how large the manufacturing and assembly deviations can be in relation to the relative position between roller and cam, you can provide correspondingly large inlet funnels at the edges of the cam. The cam flanks are slightly retracted over a certain angular range. The profile retraction is maximum at the cam edges and goes in the inlet area steadily and without corners back to 0.

The rollers are caught almost gently this way.

An animation shows this for a globoidal cam gear in the developed view.

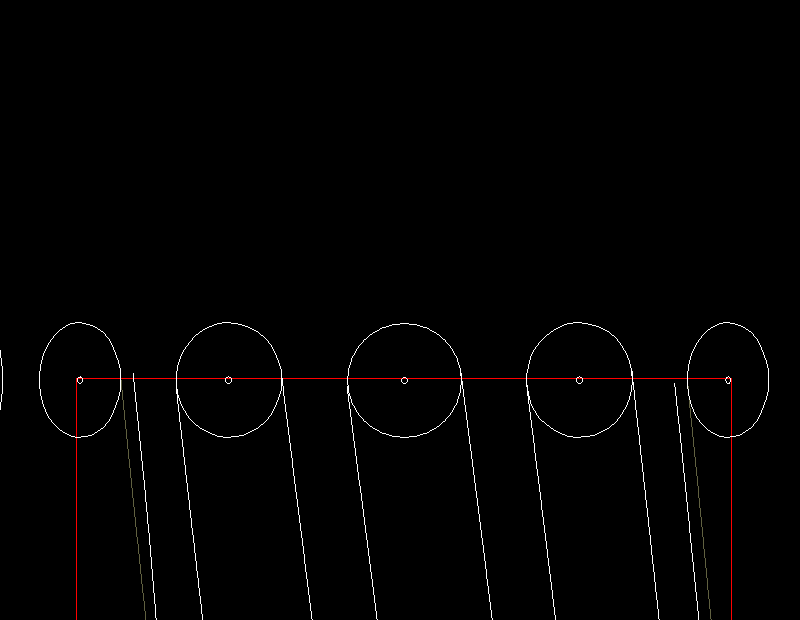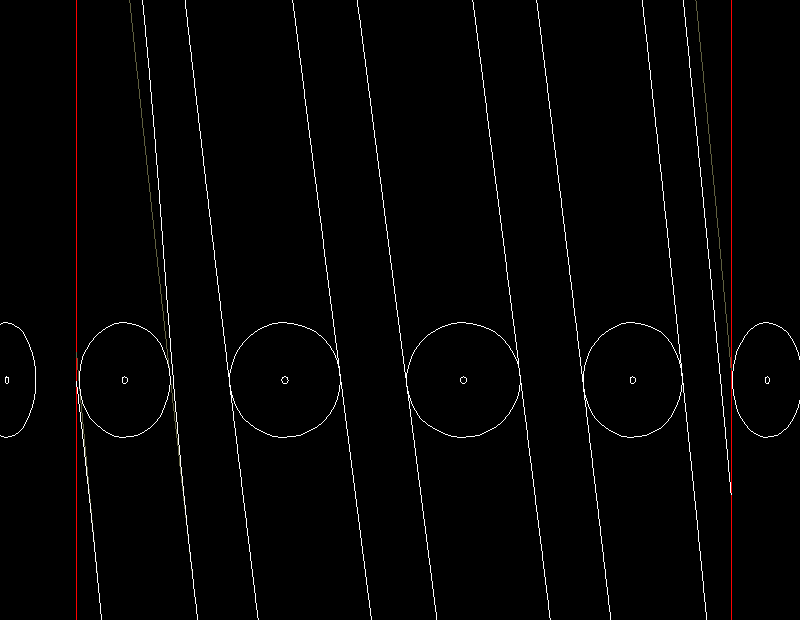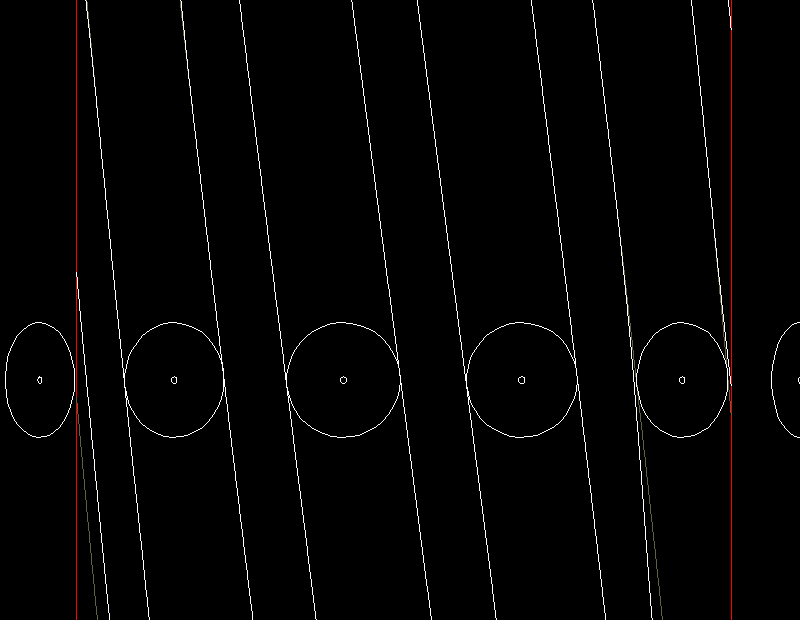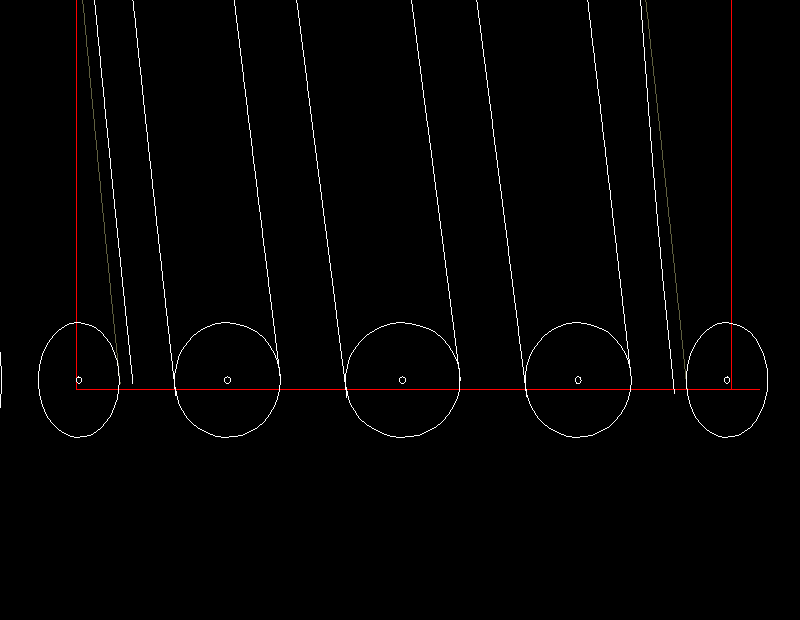
Shown in gray are the theoretical cam flanks without freecuttings, in white the actually produced cam flanks with profile retractions. The tolerance for the free movement of the roller in the groove is not taken into account here. It can be seen that the rollers lie in the middle of the cam on both flanks of the cam, while they have a significant distance at the edges of the cam.

Note: Here, too, we exaggerated the amount of the profile retraction drastically to show the effect.

Freecuttings are often combined withm spreading the cam grooves.

Cylindrical indexing cam gears also use freecutting in the entry and exit areas. Here you will find a 3D animation for that.

Back to top

Roundings

When milling globoidal cams, at the entrance and exit of the cam grooves arise acute and obtuse-angled edges, which are often rounded off in practice with the flex.

The OPTIMUS MOTUS NC postprocessors offer options for directly integrating fillets of different types and definable rounding radii into the generated NC data. The NC programs then contain not only program blocks for the production of the actual cam flank, but also the fillets.

The picture shows different rounding types that are considered.

Back to top

Chamfers

The burrs that remain when cutting the flanks of globoidal cams are often removed manually in practice with a file or flex. Despite high effort, the result is not very nice.

Corresponding postprocessors in the OPTIMUS MOTUS software generate NC data for deburring tools in order to machine deburring of the globoid cam flanks.

You get clean, uniform chamfers with a defined width. At low cost you get a high quality result.

Back to top

Track concatenation

For multiple-track indexing cams, the OPTIMUS MOTUS software automatically links the NC data of the individual tracks to a complete NC program for all tracks.

Back to top

NC Optimization

Cams for globoidal indexers are processed five-axis. The classic strategy of finishing with tool diameter = roller diameter is expensive, because it is always necessary to procure milling cutters which, depending on the size of the gear, also have to have different diameters.

Our software OPTIMUS MOTUS calculates a tool radius compensation for globoidal cams and generates NC data either directly for a defined tool diameter or - with the aid of parameter programming - for variable tool diameters. The concrete tool diameter can then be entered on the CNC machine.

This postprocessor option in OPTIMUS MOTUS saves money in tool procurement.

When cutting a globoidal cam with a standard tool theoretically too much material remains. The profile deviations increase with the slope of the cam, the flank height and the difference between roller and tool diameter. We estimate the deviations mathematically to make sure that the cam can be produced precisely enough with the given standard tool diameter.

In addition, the software numerically optimizes the NC data if desired so that the manufacturing deviations are reduced to a minimum.

Back to top

To the homepage of Nolte NC-Kurventechnik GmbH

Start Mechanisms Kinematics CamDesign Indexing Servo+PLC MotionDesign Training Software Service CamCalculation Imprint PrivacyPolicy
Variants Evaluation Spreading Freecutting Fillets Chamfers TrackConcatenation NC-optimization 

> Contact